A new method for operating a continuous-flow diffusion chamber to investigate immersion freezing: assessment and performance study

Glaciation in mixed-phase clouds predominantly occurs through the immersion-freezing mode where ice-nucleating particles (INPs) immersed within supercooled droplets induce the nucleation of ice. Model representations of this process currently are a large source of uncertainty in simulating cloud radiative properties, so to constrain these estimates, continuous-flow diffusion chamber (CFDC)-style INP devices are commonly used to assess the immersion-freezing efficiencies of INPs. This study explored a new approach to operating such an ice chamber that provides maximum activation of particles without droplet breakthrough and correction factor ambiguity to obtain high-quality INP measurements in a manner that previously had not been demonstrated to be possible. The conditioning section of the chamber was maintained at −20 ^∘C and water relative humidity (RH_w) conditions of 113 % to maximize the droplet activation, and the droplets were supercooled with an independently temperature-controlled nucleation section at a steady cooling rate (0.5 ^∘C min⁻¹) to induce the freezing of droplets and evaporation of unfrozen droplets. The performance of the modified compact ice chamber (MCIC) was evaluated using four INP species: K-feldspar, illite-NX, Argentinian soil dust, and airborne soil dusts from an arable region that had shown ice nucleation over a wide span of supercooled temperatures. Dry-dispersed and size-selected K-feldspar particles were generated in the laboratory. Illite-NX and soil dust particles were sampled during the second phase of the Fifth International Ice Nucleation Workshop (FIN-02) campaign, and airborne soil dust particles were sampled from an ambient aerosol inlet. The measured ice nucleation efficiencies of model aerosols that had a surface active site density (n_s) metric were higher but mostly agreed within 1 order of magnitude compared to results reported in the literature.

1 Introduction

Atmospheric ice nucleation plays an important role in initiating precipitation in clouds that consist of a mixture of supercooled liquid water droplets and ice crystals and in catalyzing the formation of ice particles within high-altitude cirrus clouds (Lohmann and Feichter, 2005; Boucher et al., 2013). This important step toward ice formation also affects the lifetime and radiative properties of these clouds; however, ice nucleation mechanisms are poorly understood and parameterized in cloud models (e.g., Hoose and Möhler, 2012; Murray et al., 2012; Kulkarni et al., 2012; Kanji et al., 2017; Knopf et al., 2018). Homogeneous ice nucleation is responsible for the formation of ice particles in dilute water and supercooled solution droplets at temperatures lower than ≈ −38 ^∘C (Pruppacher and Klett, 1997). Ice nucleation can also proceed through heterogeneous ice nucleation triggered by ice-nucleating particles (INPs) (Vali et al., 2015). Multiple heterogeneous ice nucleation mechanisms have been proposed, such as deposition nucleation (ice formation on INPs directly from the vapor phase), contact freezing (freezing initiated by INPs the moment they come into contact with a supercooled droplet), and condensation and immersion freezing (freezing initiated by immersed INPs within the supercooled water or solution droplets). Deposition nucleation has also been referred to as a pore condensation and freezing mechanism because it is similar to immersion freezing but occurs in pores (Marcolli 2014). Nevertheless, the immersion-freezing mode is thought to be the most important process for the formation of ice particles within mixed-phase clouds (e.g., Ansmann et al., 2009; Westbrook and Illingworth, 2013).

Immersion-freezing measurements are commonly made using continuous-flow diffusion chamber (CFDC) devices (e.g., Rogers, 1988; Chen et al., 1998; Stetzer et al., 2008; Kanji and Abbatt, 2009; DeMott et al., 2010; Friedman et al., 2011; Chou et al., 2011; Jones et al., 2011; Kanji et al., 2013; Boose et al., 2016; Garimella et al., 2016, Schiebel, 2017; Zenker et al., 2017). These chambers consist of two ice-coated parallel walls held at different temperatures, and different ice supersaturations are achieved by regulating the temperature gradient. Aerosols sampled into CFDCs are subjected to known discrete temperature and relative humidity conditions, and when the water relative humidity (RH_w) is more than a few percent above 100 %, droplets will activate on the majority of particles within the growth section of the chambers. CFDCs also typically have an evaporation section at the bottom of the chamber where the wall temperatures are controlled in order to evaporate the droplets that do not freeze. Frozen droplets are counted using an optical particle counter (OPC) to determine the atmospheric INP concentrations (Garimella et al., 2017). However, the maximum RH_w values achievable in this manner can limit the ability to determine the maximum immersion-freezing fraction (DeMott et al., 2015).

Here, we have expanded the capabilities of the CFDC-style device to achieve the maximum activation of particles to detect the immersion-freezing number concentrations of INPs at various supercooled temperatures. We present data to assess the performance from a newly developed CFDC in which all individual aerosol particles are activated to droplets, and these droplets are exposed to a sequence of supercooled temperatures. This is accomplished by modifying the existing design of the Pacific Northwest National Laboratory (PNNL) ice chamber (e.g., Friedman et al., 2011; Kulkarni at al., 2012). In the modified version, the growth section of the chamber was maintained at a higher RH_w level under moderate supercooling conditions to activate all particles to supercooled droplets, whereas the evaporation section was always held at ice-saturated conditions and cooled over a range of temperatures at a known constant rate. The evaporation section serves two purposes in this case: it induces the freezing of droplets and evaporates the unfrozen droplets. Validation experiments using standard salt solutions are presented. Various INP proxies for mineral dust types that have previously shown ice nucleation ability over a wide span of supercooled temperatures were used to test the performance of the modified chamber.

2 Experimental design and performance validation

2.1 Description of the existing and modified chamber

The PNNL CFDC-style ice chamber operated in the traditional mode, referred to as the Compact Ice Chamber (CIC)-PNNL, has been described previously (e.g., Friedman et al., 2011; Kulkarni at al., 2012). The chamber consists of two sections: a growth section and an evaporation section joined together but thermally isolated from each other. Each section consists of two parallel vertical surfaces that are coated with a thin layer of ice, and these plates are independently temperature-controlled using external cooling baths (Lauda Brinkmann Inc.). Application of an ice layer (≈ 0.5 mm thick) on these surfaces involved three consecutive steps: cooling the plates of the chamber to −25 ^∘C, filling the gap between the two parallel surfaces with deionized water (≈ 18 MΩ cm), and expelling the water after 20 s. To produce the desired water or ice supersaturation conditions, a horizontal linear temperature gradient between the plates was applied, and the corresponding temperature and RH_w or relative humidity with respect to ice (RH_ice) were calculated using the Murphy and Koop (2005) vapor pressure formulations. The single sheath and sample flow rates were 5 and 1 liters per minute (L min⁻¹), respectively, resulting in a total particle residence time of ≈ 10 s in the chamber. The temperature gradient was applied such that supersaturation conditions RH_w≈ 106 % were achieved in order to investigate the immersion-freezing efficiencies of both atmospheric and laboratory-generated INPs. An OPC (CLiMET, model CI-3100) was used to classify the particles as ice crystals if they were greater than a certain size threshold (≈ 3 µm in diameter). The ice fraction (F_ice) was calculated by using the ratio of the ice crystal concentration classified by the OPC to the total condensation nuclei (CN) concentration that entered the chamber. The CN concentration was provided by a condensation particle counter (CPC; TSI 3775). Blank experiments using dry and filtered sample air were also performed at the beginning and end of each experiment for ≈ 10 min to calculate the background number of ice particles. Further, these ice particles were subtracted from the ice crystal concentration measured by the OPC, and the F_ice was corrected.

Figure 1 shows the vertical cross-sectional geometry of the modified mode PNNL ice chamber, which is now referred to as the modified compact ice chamber (MCIC). This chamber design has a parallel-plate CFDC-style geometry, whose principle of generating a supersaturation between the two parallel surfaces and determining the F_ice is similar to that of the existing CIC chamber but with modifications as described here. The growth and evaporation sections of the MCIC chamber are now referred to as the conditioning and nucleation sections, respectively. The lengths of the two sections are identical (0.45 m), which limits the total particle residence time to ≈ 10 s. The droplet residence and nucleation times within the chamber are a maximum of 6.5 and 2 s, respectively. The chamber wall temperature values as a function of time during one typical ice nucleation experiment are shown in Fig. 2. During our study, the temperature controller of the cooling thermostat was programmed such that the warm and cold wall temperatures of the conditioning section were set to −9 and −27 ^∘C, respectively. The resulting water and ice saturation conditions are shown in Fig. 3. Here, the conditioning section temperature was chosen based on previous knowledge about the onset temperature of the INP test species being needed to induce the nucleation of ice at colder temperatures (< −20 ^∘C) and the lower detection limit being needed to measure ice concentrations for temperatures warmer than −20 ^∘C. The shaded region shows the period (≈ 30 min) of one ice nucleation measurement; i.e., the OPC data from this period only are analyzed. The F_ice now indicates the cumulative fraction of droplets frozen as a function of the decreasing temperature in the nucleation section (see Text S1 in the Supplement). This metric of reporting ice nucleation results is commonly used to report frozen fraction vs. temperature (F_ice vs. T) results (see e.g., DeMott et al., 2018; Kanji et al., 2017; Kohn et al., 2016). The isothermal conditions of the nucleation section are maintained at ice saturation and cooled at a steady rate (0.5 ^∘C min⁻¹) by a separate cooling bath in order to determine the immersion-freezing efficiency of INPs as a function of supercooled temperature. The choice of steady-state cooling rate is empirical at this moment, and the experiment is terminated when the nucleation section reaches ≈ −44 ^∘C. This additional supercooling below the onset of homogeneous freezing temperature allowed for freezing data to be obtained for use in controlling the data quality and in accounting for the uncertainty within the temperature. The implications of higher cooling rates for INP measurements were also explored. The particle residence time (≈ 5 s) and ice-saturated conditions of the nucleation section allow a sufficiently sized differential between supercooled droplets and ice crystals and in fact prevent “droplet breakthrough” (Stetzer et al., 2008). While keeping the conditioning section conditions constant, the temperature of the nucleation section is raised to ≈ −20 ^∘C to prepare for the next ice nucleation measurement. This operation allows us to probe the immersion-freezing efficiency of INPs at various temperatures (−20 to −44 ^∘C) multiple times (∼ 5) before another layer of ice coating is applied. After more than five ice nucleation measurements or after approximately 3 h, we see the reduced F_ice of standard solution droplets (discussed below). The particle pulse experiments (Garimella et al., 2017) using size-selected 300 nm mobility diameter ammonium sulfate particles and ≈ 10.5 s pulses were performed. The temperature of the conditioning and nucleation sections were held constant at 20 ^∘C, and the particle concentration at the chamber outlet using the CPC was measured. These measurements show that ≈ 16 % of total particles that enter the chamber have moved outside of the lamina (Fig. S1). Therefore, higher RH_w values are used in the chamber to activate these particles to droplets (see below). Simulations (see below) were performed to investigate the sensitivity of polydisperse particles. The particle residence times of three different monodisperse particles (0.3, 1.0, and 2.0 µm) traversing the chamber were calculated (Fig. S1). Results show that the residence times of these particles are similar, indicating that monodisperse size pulse experiments are also applicable to other size particles.

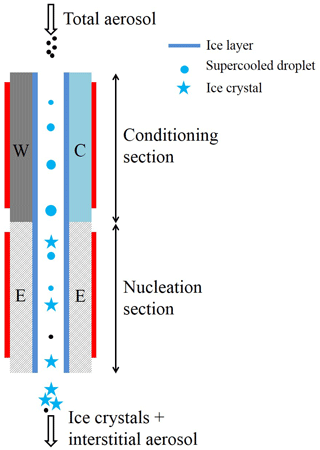
Figure 1 Schematic showing the geometry of the MCIC (expanded for clarity). INP proxies are activated to droplets within the conditioning section, and these supercooled droplets are steadily cooled within the nucleation section, from ≈ −20 to ≈ −42 ^∘C, to induce freezing of droplets and evaporate unfrozen supercooling droplets. The residence time in each section of the chamber is ≈ 5 s, and the ice layer spans both sections of the chamber. A cyclone impactor upstream of the ice chamber is used to remove the larger particles (> 1.5 µm in diameter) while sampling airborne arable dust particles. The heating tapes (red rectangular strip) are attached to the walls to precisely control the temperature of the walls. W: warm wall; C: cold wall; E: nucleation section wall. The length of both the conditioning and nucleation section is 0.45 m. The width of the chamber is 0.15 m. The gap between warm and cold walls is 0.01 m.

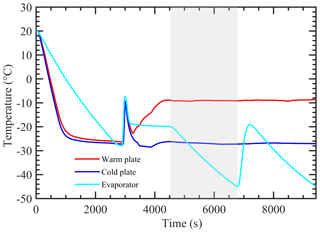
Figure 2 Measured temperature of warm, cold, and nucleation section walls during a typical experiment. The shaded area indicates the experimental conditions during one ice nucleation measurement. During this INP measurement, the temperature of both warm and cold walls is kept constant, while the nucleation section is cooled at a steady rate (0.5 ^∘C min⁻¹).

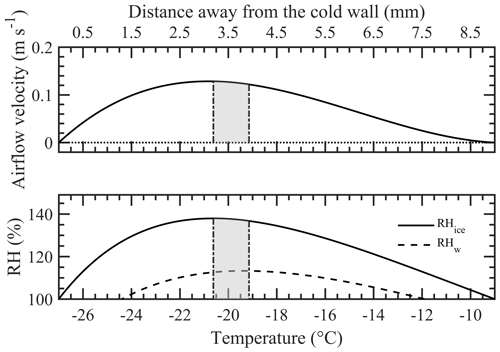
Figure 3 Steady-state airflow velocity and relative humidity (RH) conditions calculated using the mathematical model developed by Rogers (1988) within the conditioning section of the ice chamber. The chamber warm wall (left) and the cold wall (right) are at −9 and −27 ^∘C, respectively. The shaded area between the two vertical dash-dotted lines shows the boundaries of aerosol lamina under at these above temperatures and flow conditions (sheath flow: 5 L min⁻¹; sample flow: 1 L min⁻¹). The profiles are asymmetric because of the thermophoretic drift of the flow, caused by the thermal gradient between the walls, towards the colder wall. The conditioning section is always supersaturated with respect to ice (RH_ice > 100 %), and except the near-wall positions, the section is also supersaturated with respect to water (RH_w > 100 %).

2.2 Numerical modeling

At the entrance of the nucleation section, the temperature and RH_w profiles can be unsteady. To better understand the flow patterns of these profiles within the transition zone and the zone's impact on droplet behavior, numerical simulations using computational fluid dynamics (CFD) were performed. In this study, the warm and cold walls of the conditioning section were maintained at −9 and −27 ^∘C, respectively, and the nucleation section was maintained at −20 ^∘C. Analytical steady-state calculations based on Rogers (1988) were also used to understand the nature of the flow velocity profile and the position of the aerosol lamina between the warm and colder walls (Fig. 3). The results show that the operating conditions of the chamber produce a skewed velocity profile and that the aerosol lamina is displaced toward the colder wall. The aerosol lamina is surrounded by filtered sheath flow, and its width is determined by the ratio of sample to sheath airflow. Because sample flow ideally occupies this fraction of the total flow, the aerosol lamina experiences a range of temperature and saturation conditions. The center temperature and RH_w conditions, including uncertainty across the aerosol lamina (assuming ideal confinement in the lamina), are −19.7 ± 0.7 ^∘C and 113 ± 0.5 %, respectively. Additional simulations were performed to understand the center temperature and RH_w conditions required to confine the particles that are moved out of the aerosol lamina. We found that the revised uncertainties for center temperature and RH_w conditions were ±0.9 ^∘C and ±0.7 %, respectively.

CFD simulations were performed to achieve a complete description of the velocity, ice saturation, and temperature conditions within the chamber (Fig. 4a). A three-dimensional mesh of the chamber geometry was generated and exported to the commercially available CFD software ANSYS Inc (2016). The CFD software solver was the pressure-based steady-state Navier–Stokes equation, which has implicit and absolute velocity formulations. The viscous model – the standard renormalization group theory (RNG) κ–ε turbulence model – was used. This model treats velocity fluctuations better than other turbulence models for such a geometry. This turbulence model was used in conjunction with species transport modeling capability such that the effects of smaller eddies of fluid motion were better captured. The pressure outlet boundary condition was used, as were the CFD solution method to couple the pressure–velocity and the default SIMPLE scheme. The Lagrangian discrete-phase model was used to simulate the potential INP trajectories released from the sample injection region to the outlet end of the chamber. The simulations were performed using an “uncoupled approach”, which means the motion of INP particles does not influence the fluid-flow pattern. The temperature and RH_w fields of the INP trajectories were used to calculate the droplet growth and evaporation trajectories using a water vapor diffusion growth theory (Rogers and Yau, 1989) that neglects temperature corrections and kinetic and ventilation effects and assumes perfect mass and thermal accommodation coefficients (Text S2).

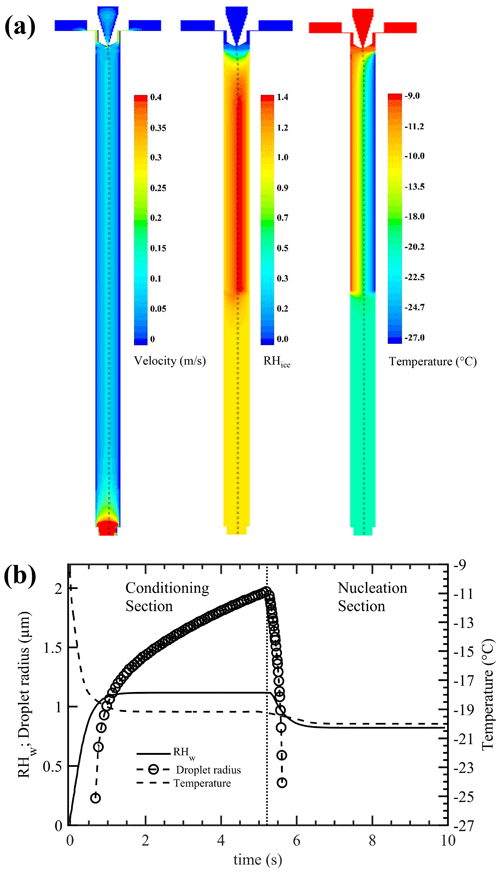
Figure 4 (a) Contours of CFD-calculated airflow velocity, RH_ice, and temperature profiles within the ice chamber. Warm and cold walls of conditioning section are maintained at −9 and −27 ^∘C, respectively. The nucleation section is maintained at −20 ^∘C. The dashed line shows the trajectory of a single INP within the aerosol lamina transiting through the chamber. (b) CFD-calculated temperature and RH_w profiles of a potential INP released from the sample injection region to the outlet end of the chamber. Analytical calculations of droplet growth and evaporation of such a potential INP (0.3 µm in diameter) are also shown. The left and right sides of the vertical dotted line represent the conditioning and nucleation sections, respectively. See the text for more details. Simulation results at other nucleation section temperatures are shown in Figs. S1–S4.

The CFD-simulated airflow velocity and RH_ice profiles from the central region of the conditioning section are nearly similar to the analytical solution (Fig. 4a). Both calculations show the presence of maximum humidity values near the middle of the chamber but slightly displaced values toward the cold wall. The fluid-flow temperature characteristics from the moment the aerosol lamina joins the sheath flow show that the aerosol sample quickly (< 0.5 s) cools at the entrance of the conditioning section. To gain a better understanding of RH_w and temperature conditions within the conditioning and nucleation sections, the simulated data set of a potential INP trajectory transiting within the chamber is shown (Fig. 4b). The potential INPs experience nearly constant RH_w and temperature conditions within a short time, ≈ 1 s, after entering the conditioning section. The potential INPs are assumed (i.e., sub-saturated particle growth is ignored) to activate to droplets because they are greater in size than cloud CN sizes (Seinfeld and Pandis, 2016) and grow as long as RH_w is increasing or remains constant. As the INPs enter the nucleation section, their RH_w and temperature values equilibrate with the nucleation section conditions. These calculations show that the droplets grow to ≈ 4 µm in diameter, and they shrink as the RH_w and temperature decrease (within the nucleation section). Note that droplet freezing within the nucleation section is not simulated in these simulations.

Additional simulations with the nucleation section temperature set to −30 and −37.5 ^∘C were also performed (Figs. S2–S5). These simulations show that the RH_w field of a potential INP slightly decreases (≈ 0.5 %) and then increases within a very short period of time (< 0.5 s) at the entrance region of the nucleation section. However, calculations show that such a perturbation does not affect the droplet evaporation behavior within the nucleation section because they all evaporate within ≈ 1 s after they enter the nucleation section and within the uncertainty limits of the set temperature of the nucleation section but not before they reach the set temperature (see Figs. S2–S4). The simulations are extended to understand the RH_w and temperature conditions of potential INPs released from different regions of the inlet section of the chamber. Simulations of five potential INPs are shown in Fig. S5. It is observed that INPs experience various temperature conditions (−17 to −19.5 ^∘C) within the conditioning section, but after ≈ 0.5 s they all enter the nucleation section, and the temperature of each trajectory is identical.

Additional evaporative cooling calculations were performed to understand the suppression of droplet temperature while they enter the nucleation section. In the nucleation section the supercooled droplets experienced sub-saturation (RH_w > 0.8) and colder temperature conditions (> −37.5 ^∘C). The Kulmala evaporative model (Su et al., 2018) was used to determine the surface temperature of these droplets using steady-state aerosol lamina airflow velocity (Fig. 3) and theoretically predicted RH_w fields (Figs. S2–S4). The calculations (Table S1) show the negligible effect of evaporative cooling on the droplet temperature such that additional supercooling is within the reported temperature uncertainty (= ± 0.7 ^∘C) across the aerosol lamina, and therefore evaporating-droplet cooling effects within the nucleation section are ignored.

2.3 Homogeneous freezing of ammonium sulfate particles

The temperature conditions within the nucleation section were validated using size-selected ammonium sulfate (AS) particles. These particles were generated by atomization of an aqueous solution made by dissolving AS (1 g) and Milli-Q water (18.2 MΩ cm; 100 g), resulting in a 1 wt % solution concentration using a constant output atomizer (TSI 3076). The atomized droplets were transported through a diffusion drier to obtain the dry particles, which were further transported to the differential mobility analyzer (DMA; TSI 3081) to obtain size-selected particles that had mobility diameters of 200 nm. The choice of this size allowed for the generation of the maximum number concentration of monodisperse particles. The concentration of these size-selected particles was measured using a CPC, and the particles were further transported to the ice chamber. As stated previously, the temperature and RH_w conditions within the conditioning section were −20 ^∘C and ≈ 113 %, respectively, and these conditions were held constant, which led to droplet activation of size-selected AS particles. Next, the nucleation section was steadily cooled from −20 to −40 ^∘C, and the ice particles exiting the chamber were classified as ice particles. The ice particle size distribution with supercooling is shown in Fig. 5a. The results show that the droplets began to freeze via a homogeneous freezing mode at ≈ −37.5 ^∘C. The maximum number of ice particle concentrations was observed at ≈ −38.5 ^∘C, when all the droplets froze. The nucleation section is always maintained at RH_ice= 100 % (see Fig. 4a), and such ice saturation conditions do not grow or sublimate the ice crystals. Therefore, ice particle size measured by the OPC can be representative of the size of the droplet while freezing. At slightly warmer temperatures (between −38.5 and −37.5 ^∘C), we observe ice particles of ≈ 2.0 µm in diameter. The appearance of these smaller ice crystals could be because of the freezing of these smaller droplets (a consequence of evaporation within the entrance zone of the nucleation section) compared to ≈ 5.0 µm droplets at ≈ −38.5 ^∘C. These homogeneous freezing threshold temperature values are in agreement with previous studies (e.g., Ignatius et al., 2016; Kohn et al., 2016). For example, Kohn et al. (2016) found 100 % freezing of supercooled dilute aqueous solution droplets at ≈ −38.2 ^∘C. Theoretical calculations using a homogeneous nucleation rate (e.g., Earle et al., 2010; Atkinson et al., 2016) were performed to predict the homogeneous freezing curves of droplets that were 4 µm in diameter. Homogeneous freezing curves for various probable droplet residence times within the nucleation section are shown in Fig. S6. Note the good agreement between the experimental and predicted freezing temperatures. These results also show the complete evaporation of supercooled droplets within the nucleation section because no ice particles are observed above −37.5 ^∘C, and therefore the freezing results (see Sect. 3) at warmer temperatures (> −37 ^∘C) can be ascribed to the heterogeneous freezing of the droplets or immersion freezing.

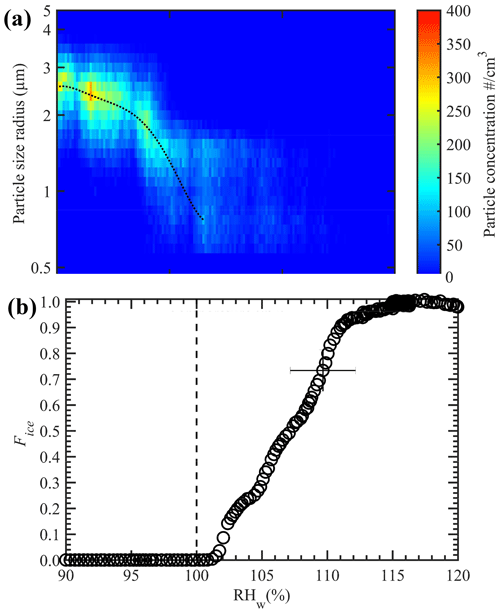
Figure 5 Homogenous freezing of water droplets containing 1 wt % ammonium sulfate solution. (a) OPC-classified ice particle concentrations as a function of ice crystal diameter at different temperatures. Warm and cold walls of conditioning section are maintained at −9 and −27 ^∘C, respectively. (b) The fraction of frozen solution droplets with RH_w, where the temperature of the nucleation section is maintained constant at −42 ^∘C to induce droplet freezing via the homogeneous freezing mode, and RH_w within the conditioning section was steadily increased from 90 % to 120 %. Slightly colder temperature (−42 ^∘C) than homogeneous freezing limit (≈ −38.5 ^∘C; panel a) is used to account for the uncertainty within temperature and RH_w conditions. The dashed line in panels (a) and (b) indicate the increase in freezing fraction of droplets trend (for illustration purposes) and the onset of saturation line, respectively. The uncertainty in RH_w is shown as an error bar (see the text for more details). The uncertainty in F_ice is 1 standard deviation (n = 3). For clarity, error bars are shown only for one data point.

This experimental setup was further applied to understand the relationship between the F_ice of AS particles relative to the RH_w conditions within the conditioning section. The aim was to investigate the RH_w value at which all the size-selected AS particles activate to droplets. Here, the nucleation section was held at −42 ^∘C to induce homogeneous freezing of solution droplets, while the RH_w within the conditioning section was steadily increased. It can be seen that RH_w values close to 113 % are required before all the AS particles are activated to droplets and measured as ice crystals (Fig. 5b). Higher RH_w values enable the encapsulation of all particles that are within and may spread outside of the width of aerosol lamina into droplets (Garimella et al., 2017). In addition, high-saturation conditions help grow the droplets to the larger size, so they survive long enough to induce the freezing of droplets within the nucleation section.

2.4 Sample preparation

The immersion-freezing efficiency of K-feldspar, illite-NX, Argentinian soil dust, and airborne soil dusts from arable region particles was measured to test the performance of the MCIC. K-feldspar (BCS376) was purchased from the Bureau of Analysed Sampled Ltd, UK. Dry-dispersed (TSI 3433) K-feldspar particles that had a mobility diameter of 400 nm were size-selected by a DMA, and the nearly monodisperse particles were transported to the CPC and ice nucleation chamber. Based on theoretical calculations (Baron and Willeke, 2001), the distribution of these classified particles may also contain sub-populations of double- (≈ 700 nm) and triple-charged (≈ 985 nm) particles. Laboratory measurements showed that the contribution of double- and triple-charged particles was less than 7 % and 3 %, respectively, which also justified the choice of 400 nm size particles. Therefore, the multiply charged particle contribution is ignored, and the K-feldspar aerosol stream is assumed to consist only of particles whose mobility diameter equals 400 nm. However, the surface area of multiply charged particles could influence F_ice because these large particles (> 400 nm) provide larger surface areas (Lüönd et al., 2010). Illite-NX and Argentinian soil dust were sampled at the AIDA (Aerosol Interaction and Dynamics in the Atmosphere) chamber facility during the Fifth International Ice Nucleation Workshop (FIN-02) campaign (DeMott et al., 2018). During the campaign, the two aerosol types were dry-dispersed in two different chambers: an 84 m³ AIDA chamber and a 4 m³ aerosol particle chamber (APC); but in this study, we sampled directly from the APC. The details of particle generation and aerosol properties are described by DeMott et al. (2018). The direct sampling of these two aerosol types corresponds to experiment numbers 8 and 10 conducted on 16 and 17 March 2015, respectively.

Airborne soil dust from an arable region or shortly airborne arable dust particles were sampled at the PNNL sampling site during a regional windblown dust event. The PNNL sampling site is located within the Columbia Plateau, WA, USA, which is confined by the Rocky Mountains to the east, the Blue Mountains to the south, and the Cascade Mountains to the west. The region was once covered with basalt lava but is now built up with loose topsoil–loess. This fine soil, which is erodible, and the agricultural dryland farming practices make this dry soil susceptible to wind erosion. The sampling was performed during one dust event on 11 May 2017, and the average temperature, humidity, and wind speed during that day were 18 ^∘C, 60 %, and 6.26 m s⁻¹, respectively. The sampling port was ≈ 9 m above the ground on the rooftop of the Atmospheric Measurements Laboratory located on the PNNL campus in Richland, WA. The airborne dust particles were drawn into the laboratory through a cyclone impactor (URG-200-30EH), which was operated at 30 L min⁻¹ to obtain a cut point diameter equal to 1.5 µm. This size-selective sampling allowed for removal of the larger particles (> 1.5 µm) and therefore helped to classify unambiguously the ice crystals larger than 3 µm using an OPC. The CN concentration of airborne arable dust particles (> 0.1 µm) was measured using a laser aerosol spectrometer (LAS; TSI 3340). The F_ice was calculated by determining the ratio of ice crystals provided by the OPC to the CN counts measured by the LAS. In parallel to INP measurements, the particles were collected on a carbon type-B film (Ted Pella Inc.; 01814-F) for scanning electron microscopy–energy dispersive X-ray spectroscopy (SEM–EDS) analysis to better understand the size distribution and composition of these airborne dust particles. The films were mounted on the C and D stages of an SKC Sioutas impactor that had 50 % cut points of 0.5 and 1.0 µm, respectively. The impactor was operated at 9 L min⁻¹, and a total of 1183 particles were analyzed. Figure S7 shows the exemplary SEM images. The images reveal that the particles are mostly composed of minerals, and the size distribution shows the mean area equivalent diameter of ≈ 0.53 µm. The input aerosol concentration of all four INP species varied from 100 to 800 cm⁻³, and the sampling duration was ∼ 30 min.

3 Results and discussion

The MCIC was operated to measure the maximum immersion-freezing fraction of INPs. The modified design allowed for the faster (≈ 30 min) accumulation of immersion-freezing data points to develop a continuous representation of the immersion-freezing behavior of INPs compared to the traditional CIC-PNNL design, in which immersion freezing was investigated at discrete temperatures. These expanded capabilities were demonstrated by measuring the immersion-freezing properties of four INP substances: K-feldspar, illite-NX, Argentinian soil dust, and airborne arable dust particles.

The measurements of the immersion-freezing properties of the four samples were investigated at temperatures between −20 and −38 ^∘C. The averaged F_ice data over ΔT= 0.25 ^∘C temperature intervals were plotted against the midpoint temperature of each bin (Fig. 6). The vertical and horizontal error bars are equal to 1 standard deviation of the F_ice measurements (n = 3) and temperature uncertainty (±0.4 ^∘C) across the nucleation section, respectively. Freezing experiments with AS solution droplets show the homogeneous freezing threshold temperature conditions being below ≈ −38 ^∘C, and therefore F_ice data points above this temperature can be attributed to the immersion-freezing mode only. Figure 6 shows that four INP materials exhibit a distribution of immersion-freezing temperatures. The F_ice of all INP species increased with decreasing temperature, consistent with many past studies (e.g., Kanji et al., 2017). The droplets containing immersed K-feldspar particles froze at the higher temperatures. The median freezing temperatures (i.e., the temperature at which 50 % of the droplets froze) of K-feldspar, illite-NX, Argentinian soil dust, and airborne arable dust particles were −25.4, −32.6, −31.4, and −31.8 ^∘C, respectively, and the difference between freezing temperatures corresponding to F_ice equal to 90 % and 10 % was approximately between ≈ 4.5 and 7.5 ^∘C for all four INP materials.

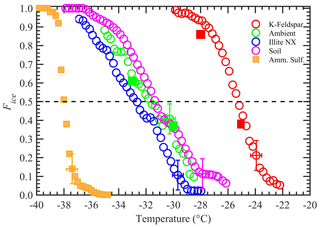
Figure 6 The F_ice of four INP test species as a function of temperature. The vertical error bar represents the 1 standard deviation of the three repeat experiments (n = 3). Temperature measurements had ±0.4 ^∘C uncertainty. For clarity, error bars are shown for only one data point. Solid square orange markers represent the freezing temperatures of water droplets containing 1 wt % AS. Other solid square markers represent data collected when the chamber was operated in a steady-state temperature mode (instead of steady cooling). The dashed horizontal line represents 50 % F_ice.

Additional experiments were performed to confirm that the dynamic temperature conditions (steady-state cooling) of the nucleation section do not affect the freezing behavior of particles. The measurements were conducted on K-feldspar and airborne arable dust particles that were prepared as described above in the sample preparation section. The temperatures of the warm and cold walls of the conditioning section were maintained at −9 and −27 ^∘C, respectively, and the nucleation section temperature was held constant (instead of steady-state cooling). The immersion-freezing fraction data points of these two species are shown as solid symbols in Fig. 6. Good agreement with the results obtained from MCIC was observed, which suggests that the temperature-ramping operation of the nucleation section (0.5 ^∘C min⁻¹) does not affect the performance of INP activation experiments. The experiments with higher cooling rates (2.5 and 7.0 ^∘C min⁻¹) had negligible effects on F_ice of airborne arable dust species (Fig. S8). Further, the time-dependent immersion-freezing framework (e.g., Vali and Snider, 2015; Herbert et al., 2014) suggests that the rapid cooling of the droplets could shift the cumulative ice fraction toward the colder temperature based on the cooling rate and particular INP material constant. However, these input parameters for the time-dependent model are not available currently to quantify the temperature shift for the present experimental conditions. Future studies that involve collocated direct and post-processing INP instruments would be needed.

These F_ice measurements were further analyzed using the ice nucleation active site density (n_s) approach that allows a comparison with other studies (see below). This approach also allowed us to compare results directly with literature data obtained using different experimental setups and various direct and post-processing INP instruments and particle generation methods. The n_s indicates the cumulative number of ice active sites that are present per unit area of particle surface and that induce nucleation of ice upon cooling from 0 ^∘C to experimental temperature T. In this calculation, time dependence is neglected, and it is assumed that the different active sites present within the droplets are responsible for the nucleation of ice. The n_s calculation follows Hiranuma et al. (2015) and DeMott et al. (2018):

$$\begin{array}{}\text{(1)}& n_{\mathrm{s}}\left(T\right)={\displaystyle \frac{-\ln \left(\mathrm{1}-F_{\mathrm{ice}}\right)}{A}}\approx {\displaystyle \frac{F_{\mathrm{ice}}}{A}},\end{array}$$

where A is the surface area per particle, and the approximation is valid for F_ice ≪ 1.0 in Eq. (1). For K-feldspar and airborne arable dust particle analysis, the surface area is calculated assuming the particles are spherical, and this assumption may overestimate the n_s; therefore, calculations should be viewed as the upper estimates of n_s. The size distribution and CPC concentrations were used to calculate the A of individual airborne arable dust particles, as described by Niemand et al. (2012). For illite-NX and Argentinian soil dust particles, the A was obtained from the FIN-02 data archive (DeMott et al., 2018). The error in n_s (Eq. 1) was calculated using the error propagation method based on the uncertainties in the F_ice and A.

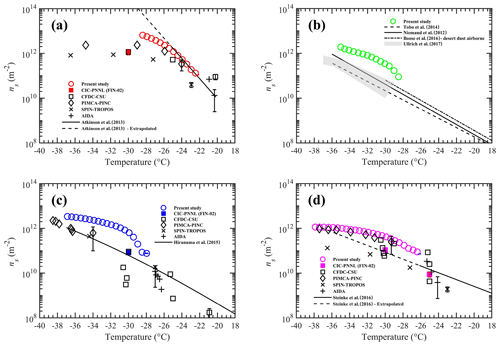
Figure 7 Ice nucleation active site density (n_s) as a function of temperature for four INP test species tested in this study. The panels (a) to (d) show n_s densities for K-feldspar, airborne arable dust, illite-NX, and Argentinian soil dust, respectively. Solid and dash-dotted lines represent various parameterizations from the literature. See the text for details. Dashed lines in panels (a) and (d) indicate the extrapolated data calculated outside the temperature limits recommended in these n_s parameterizations. The black color symbols represent n_s values from various other instruments that participated in FIN-02 activity (DeMott et al., 2018). Filled color symbols show the data from the CIC-PNNL chamber but operated at steady-state temperature and RH_w= 106 % conditions at FIN-02. For clarity, confidence intervals are shown only for one data point from each study.

Figure 7 shows n_s for the four INP materials tested in this work in comparison to parameterizations reported in previous studies. The n_s for K-feldspar is compared to the fit published by Atkinson et al. (2013). There is a good agreement with our measurements for temperatures warmer than −26 ^∘C. Atkinson et al. (2013) used a droplet-freezing cold stage technique, in which a known amount of K-feldspar material was present in each droplet sized between 14 and 16 µm. These droplets were cooled at a rate of 1 ^∘C min⁻¹, and droplet-freezing temperature data were used to construct the n_s parameterization. Note that the n_s fit from Atkinson et al. (2013) is valid up to −25 ^∘C. In our work, we extrapolated the fit outside this limit to colder temperatures for comparison. However, such linear extrapolation to colder temperatures may not be correct because, as both Niedermeier et al. (2015) and DeMott et al. (2018) (the latter from the FIN-02 campaign) have shown, the n_s values level off at temperatures colder than −25 ^∘C. The n_s for airborne arable dust was compared with the previous studies. Niemand et al. (2012) derived the n_s fit using combined immersion-freezing data from various natural dusts (Asian soil dust, Canary island dust, Saharan dust, and Israel dust). Recently, Ullrich et al. (2017) developed n_s parameterization using immersion-freezing n_s densities of various arable dusts (Saharan desert dust, Asian desert dust, Israel desert dust, Canary Island dust) for the temperature range from −14 to −30 ^∘C. Tobo et al. (2014) investigated the INP abilities of agriculture soils dusts collected from Wyoming, USA. Boose et al. (2016) investigated the INP efficiencies of airborne dust samples from four locations (Crete, Egypt, Peloponnese, and Tenerife) and generated the minimum to maximum bounds of n_s from −29 to −37 ^∘C. The comparison of our results with these previous results shows good agreement within 1 order of magnitude at colder temperatures, but the data diverge at warmer temperatures. This could be the consequence of a particularly active soil dust present in the local region. The n_s for illite-NX was compared to that of Hiranuma et al. (2015), who combined immersion-freezing data from several direct-processing INP methods to develop an n_s parameterization. Here, we used the Gumbel cumulative distribution linear fit parameters derived from dry dispersion measurements to generate the n_s fit. Our data agree within 1 order of magnitude at warmer (−28 to −30 ^∘C) and colder temperatures (−34 to −38 ^∘C), but at other temperatures (−30 to −34 ^∘C) the data diverge. Finally, we compared our data with n_s parameterization from Steinke et al. (2016). Steinke et al. (2016) used immersion-freezing data from four soil dust samples (Mongolian soil, Karlsruhe soil, German soil, and Argentinian soil) to produce an n_s fit that is valid over a temperature range between −26 and −11 ^∘C. We extrapolated the n_s fit toward colder temperatures, and the comparison shows higher n_s values but overlaps within the order of magnitude with others.

Figure 7a, c, and d also show the n_s results reported by five different direct-processing INP instruments used in the FIN-02 campaign (DeMott et al., 2018). Our data for K-feldspar nearly align with the others at warmer temperatures (> −28 ^∘C). For the illite-NX sample, agreement with the PIMCA–PINC (Portable Immersion Mode Cooling chAmber–Portable Ice Nucleation Chamber) method is within 1 order of magnitude, but the agreement is observed within 2 orders of magnitude with others. The present data for Argentinian soil dust align with the PIMCA–PINC method and agree with the others within 1 order of magnitude. The discrepancy between our results and others' could be attributed to the different capabilities employed by individual measurement methods to investigate the immersion-freezing properties. The experimental uncertainties (e.g., ice crystal detection limit, RH, and temperature error limits) from these methods could also influence the n_s results. Previously, evaporative freezing by contact nucleation inside-out has been hypothesized to explain the higher freezing temperatures and rates of ice formation observed during droplet evaporation (Durant and Shaw, 2005). Durant and Shaw (2005) showed that water droplets containing individual insoluble INPs freeze at a higher temperature compared to the immersion-freezing mechanisms. We cannot rule out that the evaporative freezing mechanism may be occurring in our experiments, and it would be responsible for the higher n_s values to be compared to those of other studies. The comparison with the CIC-PNNL chamber showed that our data agree within 1 order of magnitude. Note that CIC-PNNL (PNNL ice chamber but operated in a traditional mode; Friedman et al., 2011; Kulkarni et al., 2012) was operated at RH_w= 106 %, and its operation limited investigating immersion freezing on the entire particle population. It can be observed that for illite-NX and Argentinian soil dust samples a correction factor of 4 to 5 is needed to apply to the CIC-PNNL data to match with the data from the MCIC.

Our results can guide design considerations for future CFDC-style ice chambers. The length of the conditioning section can be increased so that higher RH_w values would not be necessary to activate all the particles to sufficiently large droplet sizes (≈ 2 µm in diameter). This design feature could help to increase the lifetime of the ice layer. Based on CFD results (Figs. S3–S5), the minimum evaporation or nucleation length required is 0.2 m. In addition, by implementing a separate refrigeration system to independently cool the nucleation section, the new operation mode presented here can be adapted.

4 Conclusions

An alternative method of operating a CFDC-style ice chamber – the MCIC – was explored to determine the immersion-freezing ability of INPs. This new mode of operation allowed us to obtain maximum immersion-freezing fractions of INPs without droplet breakthrough ambiguity. Here, instead of investigating immersion freezing at discrete temperatures, immersion freezing was investigated by activating particles to droplets at high RH_w followed by steady cooling under imposed ice-saturated conditions. The chamber performance was evaluated by testing the ice nucleation ability of four INP materials: K-feldspar, illite-NX, Argentinian soil dust, and airborne arable dust particles. In addition, we performed CFD simulations to evaluate flow, humidity, and temperature performance. The results indicate that these three thermodynamic conditions are locally fully developed, which confirms constant mass and thermal flux and therefore steady operating conditions within the chamber. Tests using size-selected AS particles showed that homogeneous freezing of solution droplets occurs in agreement with theory and previous study results and that, to activate all the particles to droplets, high RH_w values of ≈ 113 % are needed. Analytical and CFD calculations indicate that such high values are needed to grow the droplets to larger sizes so that they can survive long enough to induce freezing and to allow the particles that may have escaped the aerosol lamina to activate into droplets. Tests using the four INP materials demonstrated the activation of all individual particles to generate immersion-freezing spectra in terms of F_ice and n_s. Experimental results indicate that K-feldspar minerals induced detectable ice formation at ≈ −22 ^∘C, and maximum F_ice (= 90 %) was observed at −28 ^∘C. The other three samples induced nucleation of ice at temperatures colder than −26 ^∘C, and their maximum F_ice (= 90 %) was observed to be ≈ −36 ^∘C. The F_ice was normalized using particle surface area to calculate the n_s, and these n_s calculations show that our results are comparable to the parameterizations and data reported in the literature. We find that the majority of our n_s results are higher within 1 order of magnitude than others. Analysis of such high-temporal-resolution immersion-freezing measurements could offer better insights into the freezing properties of INPs, thereby moving us toward improved representations of the immersion-freezing ability of INPs for cloud models.